Joceline Lega
Instability of Pulse-like Deformations of an Elastic Filament
Introduction
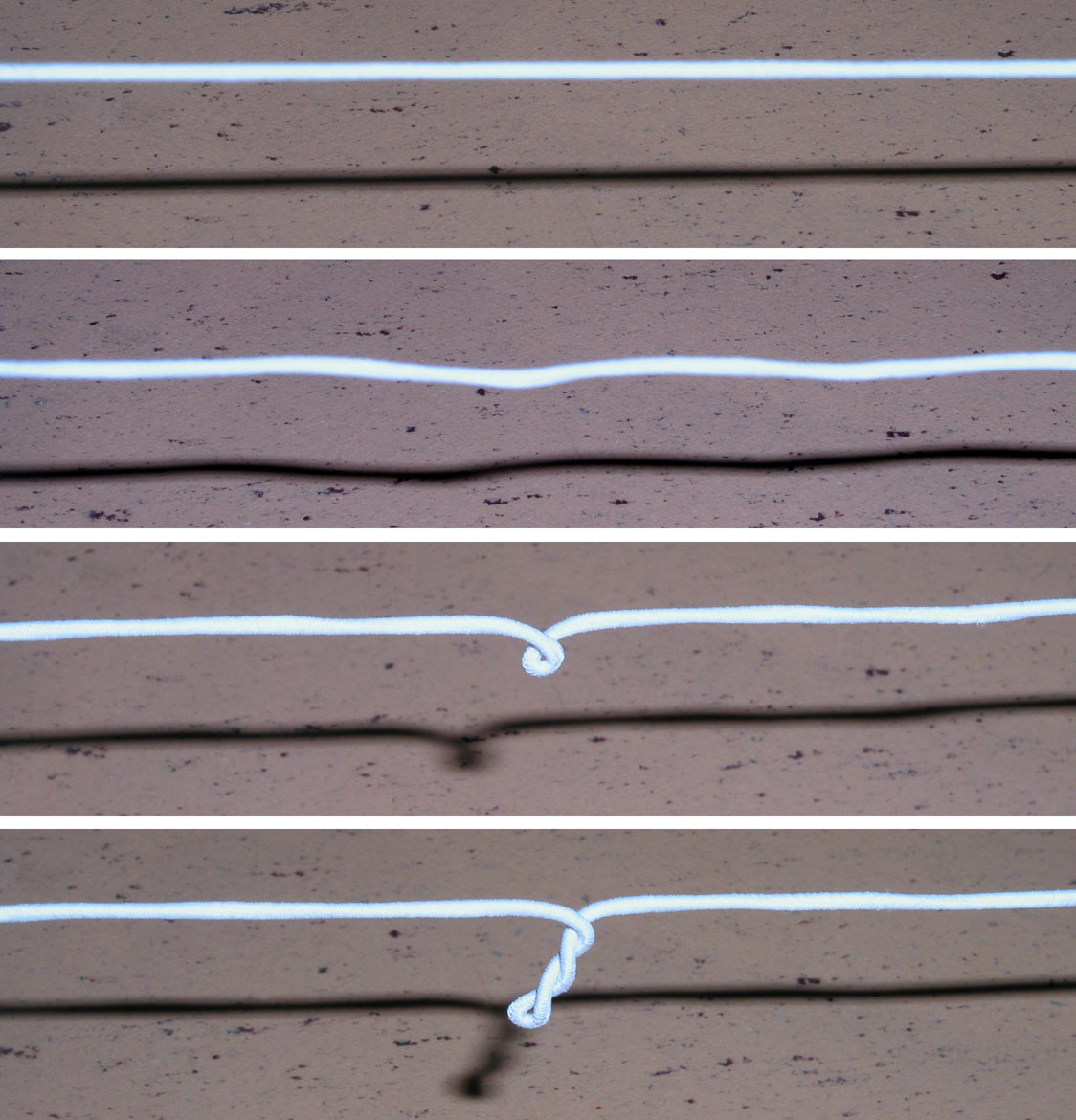 An elastic filament kept under tension and subject to increasing
twist, will undergo a series of bifurcations, as illustrated in the series of photographs
shown on the right. Near the threshold of the first bifurcation (which occurs between the
first and second panels on the right), the dynamics of the elastic filament may be
described in terms of nonlinear coupled Klein-Gordon equations [1]. These equations rule
the dynamics of the slowly varying twist and slowly varying amplitude of the helical
mode which becomes unstable at the bifurcation threshold.
An elastic filament kept under tension and subject to increasing
twist, will undergo a series of bifurcations, as illustrated in the series of photographs
shown on the right. Near the threshold of the first bifurcation (which occurs between the
first and second panels on the right), the dynamics of the elastic filament may be
described in terms of nonlinear coupled Klein-Gordon equations [1]. These equations rule
the dynamics of the slowly varying twist and slowly varying amplitude of the helical
mode which becomes unstable at the bifurcation threshold.
It was shown in [2] that the coupled Klein-Gordon equations admit a two-parameter family of particular solutions, which correspond to pulse-like deformations of the filament [2]. Numerical simulations [2] (see the figure below for the evolution of a stable pulse) indicate that these solutions may be stable or unstable, depending on the speed at which they propagate, and on the frequency at which the corresponding filament rotates upon itself.

Instability Criterion, using the Evans Function
For non-rotating filaments, one can show by means of Evans function techniques [3], that pulses are unstable if their speed lies in a prescribed interval. The Evans function [4-8] (for a review see [9]), is a function of the spectral parameter, which vanishes on the point spectrum of a linear operator. The analysis described in [3] relies on the use of a classical parity argument, which guarantees that the Evans function has to vanish at least once on the positive real axis. One can indeed show that the Evans function is real for real values of the spectral parameter, and that it is continuous on the positive real axis. Then, knowledge of the behavior of the Evans function near the origin (typically obtained by means of asymptotic expansions), together with information on the sign of the Evans function for large values of spectral parameter, leads to a sufficient condition for the existence of a zero of this function on the positive real axis. This condition in turn implies the existence of a real positive eigenvalue of the linearized operator obtained by linearizing the coupled Klein-Gordon equations about a pulse solution. This gives the instability criterion found in [3].
Spectral Stability Criterion, using Hamiltonian Techniques
One can obtain a more general instability criterion, which applies to both rotating and non-rotating filaments, by using Hamiltonian techniques. The coupled Klein-Gordon equations are indeed Hamiltonian [2], and general results [10-11] for the orbital stability of solutions to Hamiltonian systems with symmetries can be found in the literature. The theorems of [10-11] however do not directly apply here because the continuous spectrum of the linearization about a pulse solution is the whole imaginary axis, and therefore contains the origin. One can however modify the results of [10-11] in order to obtain a necessary and sufficient condition for the spectral stability of both types of pulses [12]. The instability criterion hence obtained generalizes the results of [3].
PowerPoint Presentation
Instability of Local Deformations of an Elastic Filament
References
- A. Goriely & M. Tabor, Nonlinear dynamics of filaments II: Nonlinear analysis, Physica D 105, 45-61 (1997).
- J. Lega & A. Goriely, Pulses, fronts and oscillations of an elastic rod, Physica D 132, 373-391 (1999).
- S. Lafortune & J. Lega, Instability of local deformations of an elastic rod, Physica D 182, 103-124 (2003).
- J.W. Evans, Nerve axon equations. IV. The stable and unstable impulse, Indiana Univ. Math. J. 24, 1169-1190 (1975).
- C.K.R.T. Jones, Stability of the travelling wave solution to the FitzHugh-Nagumo equation, Trans. AMS 286, 431-469 (1984).
- E. Yanagida, Stability of fast traveling pulse solutions to the FitzHugh-Nagumo equations, J. Math. Biol. 22, 81-104 (1985).
- J. Alexander, R. Gardner & C. Jones, A topological invariant arising in the stability analysis of travelling waves, J. Reine Angew. Math. 410, 167-212 (1990).
- R. Pego & M. Weinstein, Eigenvalues, and instabilities of solitary waves, Phil. Trans. R. Soc. London A 340, 47-94 (1992).
- B. Sandstede, Stability of travelling waves, in Handbook of Dynamical Systems II: Towards Applications, pp. 983-1055, B. Fielder Ed., Elsevier, 2002.
- M. Grillakis, J. Shatah and W. Strauss, Stability theory of solitary waves in the presence of symmetry, I, J. Functional Analysis 74, 160-197 (1987).
- M. Grillakis, J. Shatah and W. Strauss, Stability theory of solitary waves in the presence of symmetry, II, J. Functional Analysis 94, 308-348 (1990).
- S. Lafortune & J. Lega, Spectral stability of local deformations of an elastic rod: Hamiltonian formalism, SIAM J. Math. Anal. 36, 1726-1741 (2005).
Acknowledgments
This material is based upon work supported by the National Science Foundation
under Grant No DMS0075827.
Any opinions, findings, and conclusions or recommendations expressed in
this material are those of the author(s) and do not necessarily reflect the views
of the National Science Foundation.