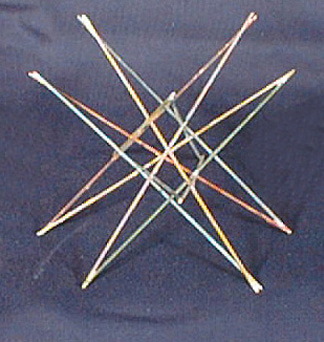

95 Schläfli’s double-six This is probably model 365 in Baker’s catalog, the “double six from [Clebsch’s Diagonal Surface]”. Schläfli’s double-six is a configuration of 12 lines and 30 points, with each point meeting two lines and each line meeting five points. The 12 lines may be divided into two groups of 6 such that no two lines in the same group intersect each other, and each line intersects exactly five lines of the other group. Schläfli’s double-six has an interesting relation with cubic surfaces. Choose nineteen points, distinct from the points in the configuration, so that four of the chosen points lie on one of the lines, and three lie on each of the five lines intersecting that line. There is, in general, a unique cubic surface through those nineteen points. Each of the 12 lines in the configuration lies on this cubic surface, since it intersects the surface in four points. Furthermore, one can construct fifteen more lines on the cubic surface by taking appropriate intersections of planes generated by the lines in the configuration, for a total of 27. If the attribution to Baker is correct, the cubic surface in this case is Clebsch’s diagonal surface, defined in four-space by the equations x^3 + y^3 + w^3 + 1 = 0 and x + y + w+ 1 = 0. |